Demos: 1S-30 Scotch Yoke Model

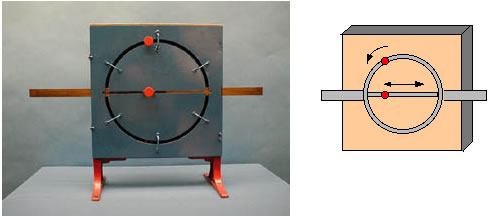
This demonstration illustrates how SHM arises from the projection of uniform circular motion onto a linear axis. The mechanism is such that the red knob on the horizontal bar always is directly beneath the red knob that is moving in a circle. Thus, the horizontally moving knob can be construed as the projection onto the horizontal axis of the knob that is moving in a circle.

Directions: Turn the crank at the rear of the apparatus as smoothly as possible to achieve uniform circular motion.

Suggestions for Presentation: Tell the students to imagine a broad source of light shining vertically downward on the apparatus. The “shadow” of the rotating knob would then be the horizontally moving knob. This introduces the idea of “projection” onto an axis. Then show mathematically that the motion along the horizontal axis is just the projection of the circular motion.

Applications: Any situation in which the projection of circular motion is onto a linear axis. For example, the Demo 1S - 18 (Turntable Oscillator) makes use of this mechanical arrangement to produce sinusoidal wave patterns.

Add to Cart | View Cart